How To Do Interior And Exterior Angles
Sum Of Interior Angles Is Interior Angle Exterior Angles Angles Sum
Remote Exterior And Interior Angles Of A Triangle Interior And Exterior Angles Teaching Geometry Exterior Angles
Problem Solving With Angles Of Polygons Mr Mathematics Com Exterior Angles Interior And Exterior Angles Interior Design Website
Understanding Interior And Exterior Angles In Triangles Interior And Exterior Angles Geometry Lessons Teaching Geometry
Pin On Homedecor
Polygon Interior Angle Sum Exterior Angle Sum Theorems Doodle Notes Interior And Exterior Angles Exterior Angles Graphic Organizers
Each vertex of the polygon corresponds to an interior angle and an exterior angle both of which are.
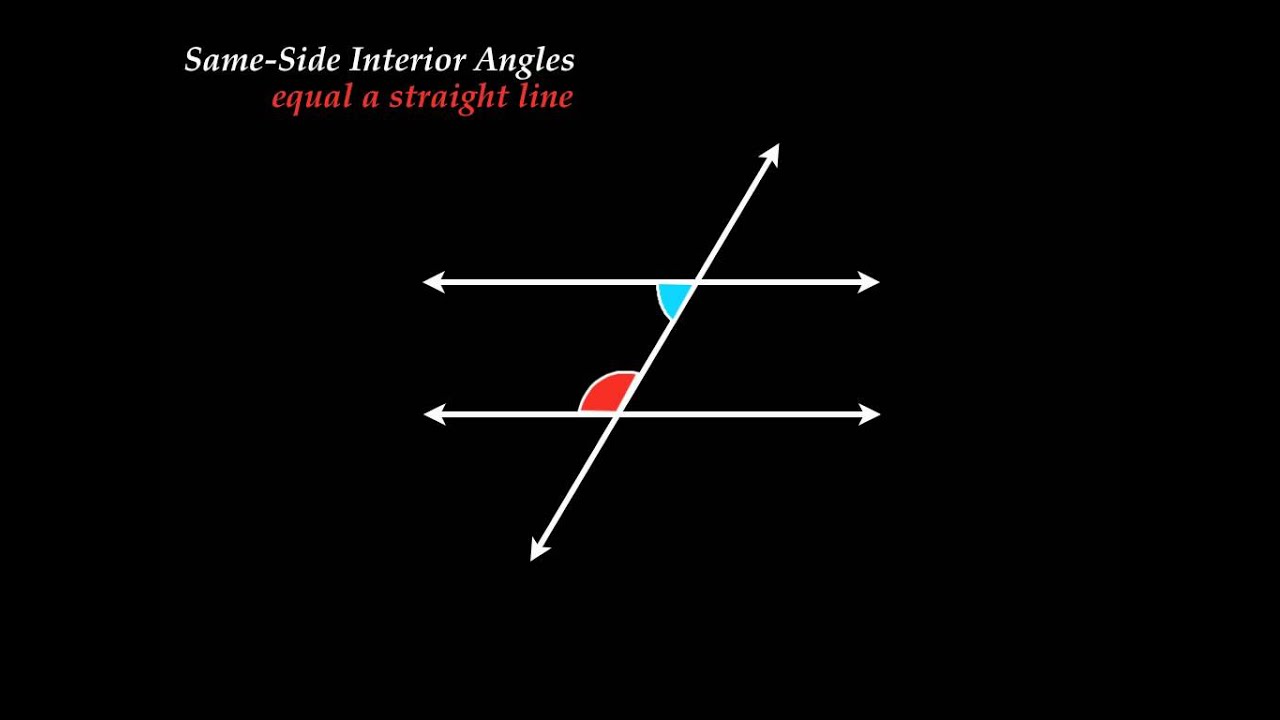
How to do interior and exterior angles. A b c 180. 1 Count by ones forwards backwards from various starting points between 1 and 100. When we add up the Interior Angle and Exterior Angle we get a straight line 180.
As a result every angle is 135. 1 Know numbers before and after a given number up to 100 1 Identify and continue patterns 2 Count from 0 by 2s 5s and 10s to a. The interior angles of a triangle add to 180.
Here is the formula. The formula for the sum of that polygons interior angles is refreshingly simple. Each Interior Angle 180n-2n Let us apply this formula to find the interior angle of a regular pentagon.
The exterior angle is 360 5 72. D a b. To find the exterior angle we simply need to take 135 away from 180.
Understanding alternate interior and exterior angles begins with becoming familiar with what is known as a Transversal Line. The opposite sides are parallel. That is the interior angle is that arc that is constituted by the intersection of two sides of the polygon being located within it.
I 8 - 2 x 180 i 1080 To find the individual angles of this regular octagon we just divide the sum of interior angles by 8. We discuss regular and nonregular. An exterior angle of a polygon is an angle outside the polygon formed by one of its sides and the extension of an adjacent side.
Angles And Polygons Interior And Exterior Angles Exterior Angles Interior Design School
Same Side Interior Angles And Same Side Exterior Angles Exterior Angles Exterior Siding Interior And Exterior Angles
Geometry Non Central Angles Interior And Exterior Angles And Secant And Tangent Relationships O Interior And Exterior Angles Teaching Geometry Math Geometry
Awesome Interior Angles Of A Triangle And Review Exterior Angles Interior And Exterior Angles Angles
Interior And Exterior Angles Of Polygons Teaching Resources Interior And Exterior Angles Exterior Angles Angles Worksheet
Exterior Angles Of A Triangle Inquiry Activity By Math Giraffe Tpt In 2021 Interior Design School Boutique Interior Design Interior Design Colleges
Proofs Without Words Interior And Exterior Angles Exterior Angles Triangle Math
Polygons Graphic Organizer And Practice Middle School Math Geometry Graphic Organizers Geometry Worksheets
Maths Mathematics Lessons Resources Lessonplans Bundles Keywords Helpful Tes Tpt Exterior Angles Interior And Exterior Angles Interior Design School
Math By Tori Triangles Unit Interior Angle Sum And Exterior Angle Remote Interior Angles Exterior Angles Theorems Math
Geometry Non Central Angles Interior And Exterior Angles And Secant And Tangent Relation Exterior Angles Interior And Exterior Angles Interior Design School
Pin Oleh Towice Di Exterior Angle Theorem
Three Proofs That The Sum Of Angles Of A Triangle Is 180 Interior And Exterior Angles Geometry Proofs Exterior Angles
